Stoichiometry

2.1 Introduction

Stoichiometry is the calculation of quantities in chemical equations. In a given chemical reaction, stoichiometry tells us what quantity of each reactant we need in order to get enough of our desired product. Because of its real-life applications in chemical engineering as well as research, stoichiometry is one of the most important and fundamental topics in chemistry.

The most simple stoichiometric problem will present you with a certain amount of a reactant and then ask how much of a product can be formed. Here is a generic chemical equation:

2 X + 2Y ---> 3Z

Here is a typically-worded problem: Given 20.0 grams of X and sufficient Y, how many grams of Z can be produced?

It is required to get familiar with the use molar ratios, molar masses, balancing and interpreting equations along with conversions between grams and moles.

 

The meaning of a chemical equation

Chemical equations give information in three major areas:

• They tell us what substances are reacting (those being used up) and what substances are products (those being made)
• The coefficients of a balanced equation tell us in what ratio the substances react or are produced
• The relative amounts of all substances involved in the reaction

2.2 Understanding chemical formulas and equations

One of the most ‘mysterious’ parts of chemical engineering is the knowledge of writing chemical formulas and equations. It is almost magical that a chemical engineer hears the name of a substance or chemical reaction and can instantly translate this into a chemical formula or equation. The depth of knowledge possessed by a chemical engineer is beyond the scope of this course and manual. But we shall definitely make a beginning at unraveling the mystery of this section of chemical engineering.

A chemical reaction takes place between two or more chemicals. But it is not so in every case. For example if zinc and iron are placed together nothing happens. Even when heated to high temperatures the two metals merely fuse and don’t react chemically. It is therefore important to understand how and why chemical reactions take place.

In their quest to make gold out of lead yester-year alchemists discovered that a certain type of compound (or element) reacted with only another specific type of compound (or element). Gold was considered pure because it has virtually no reaction with any compound under standard conditions. On the other hand even if a tiny bit of sodium comes into contact with water there is a vigorous and often explosive reaction. One conclusion that was arrived at was that the reaction took place even when extremely minute quantities of two reactive substances came into contact with each other. In other words the reaction was independent of the quantity of reactants. The term used today to describe the smallest part of an element (pure substance) is the ‘Atom’.

The first reference to this particle is found in the Vedic Period in India (around 1500 BC) where the learned sage Maharshi Kanad propounded the idea of ‘Anu’ or the smallest particle of matter that can exist independently.

The term atom was first used by the Greek philosopher Democritus and in Greek it means ‘indivisible’. Later the works of John Dalton (1808), William Crookes (1878), JJ Thomson (1879) further defined the structure of the atom.

But it was the work of Rutherford and Neils Bohr that gave rise to the structure of the atom that is in use even today. Although science has since discovered ‘sub-atomic’ particles the principles of the Rutherford-Bohr model have remained unchanged.

In chemistry it is believed that a chemical reaction takes place at the atomic level. To understand a chemical reaction and to classify elements based on their reactivity a basic understanding of the structure of the atom is necessary.

2.2.1 The structure of the atom

Rutherford conducted exhaustive experiments to determine the structure of the atom and was the first person to actually propose a model of an atom. He based his research on William Crookes’ discovery of charged particles that were called electrons and on JJ Thomson’s model of an atom. Since he found that both theories proposed by his predecessors were flawed he proposed his own model of an atom based on the experiments he conducted. The salient feature of his model was the theory that an atom consisted of a nucleus of positively charged protons with negatively charged electrons revolving around it. Hence an atom was thought of having charged particles but remained electrically neutral.

In 1913 Neils Bohr, a Danish physicist discovered that the laws of mechanics and electrodynamics could not be used to substantiate Rutherford’s theory and hence was flawed. He then suggested that electrons revolving around the nucleus were doing so in fixed orbits (also known as shells or energy levels). It is this basic information that revolutionized the way a chemical reaction was viewed.

The model of an atom proposed by Bohr details many aspects which are beyond the scope of our study and the relevant ones are used to describe a chemical reaction.

The basic structure of an atom was thought to be that of a central nucleus consisting of positively charged particles called protons surrounded by negatively charged particles that revolved at very high speed around the nucleus and were known as electrons. It was calculated that the mass of an electron was negligible when compared to the mass of an atom. It was further believed that to sustain stability and remain stationery a proton had to have higher mass. Since an atom was always electrically neutral the number of protons always equaled the number of electrons in an atom. But this theory was soon dismissed because when the system of a.m.u. (atomic mass unit) was used to calculate the mass of an atom the results didn’t add up. It was experimentally determined that the mass of the protons was far less than the total weight of the atom. The difference in mass was explained by proposing that within the nucleus there had to be present particles that had the same mass as that of protons but had no electric charge. These particles were called Neutrons.

To begin understanding a chemical reaction we need to know the three most important parts of the atom that have just been referred to along with their properties, symbols and electric charge. The table below illustrates all of these.

Sub-atomic particle	Definition	Symbol
Electron	A particle that revolves around a central nucleus; has negligible mass; has a negative charge of 1.	e
Proton	A particle making up a nucleus; has a mass of one hydrogen atom; has a positive charge of 1.	p
Neutron	A particle making up a nucleus; has a mass almost equal to one hydrogen atom; has no charge	n

The arrangement of these three sub-atomic particles gives rise to unique elements. It can therefore be said that all elements consist of these particles but acquire their uniqueness from the arrangements of these particles in their respective atoms.

The number of protons in the nucleus of an atom is called the Atomic Number and is denoted by the letter ‘Z’. Based on the fact that an atom is electrically neutral it follows that the number of protons is equal to the number of electrons present in the atom.

The number of protons and neutrons present in the nucleus of an atom are called the Mass Number and is denoted by the letter ‘A’.

Based on the above information it follows that

• Z = p = e
• A = n + p

The arrangement of electrons revolving around a nucleus is of prime importance and is based on the theory put forward by Bohr and Bury. The salient features of this theory are detailed below.

• The maximum number of electrons that can be present in any shell of an atom is given by the formula 2n2 where n = the number of the shell (counted from its proximity to the nucleus. E.g. the shell closest to the nucleus is numbered 1, the next one is 2 and so on).
• The outermost shell of an atom cannot have more than 8 electrons and the last but one shell cannot have more than 18 electrons
• Each shell or orbit of an electron need not wait to complete the maximum number of electrons permissible by the formula 2n2 before another shell is formed. It is observed that a new shell is formed as soon as the outermost shell attains 8 electrons.
• An atom stops reacting chemically once the outermost shell acquires 8 electrons or it has only one shell containing 2 electrons (as in the case of Helium).

Figure 2.1
Structure of an atom

Based on the information we can now tabulate the number of electrons in each shell or orbit for a given element. The table below lists some of the elements commonly used.

Element	No. of neutrons n = A – Z	No. of protons Z = p	No. of electrons Z = e	Electronic configuration
Hydrogen 1H1	1 – 1 = 0	1	1	1,
Helium 2He4	4 – 2 =2	2	2	2,
Carbon 6C12	12 – 6 = 6	6	6	2,4
Nitrogen 7N14	14 – 7 = 7	7	7	2,5
Oxygen 8O16	16 – 8 = 8	8	8	2,6
Neon 10Ne20	20 – 10 = 10	10	10	2,8
Sodium 11Na23	23 – 11 = 12	11	11	2,8,1
Aluminum 13Al27	27 – 13 = 14	13	13	2,8,3
Sulphur 16S32	32 – 16 = 16	16	16	2,8,6
Chlorine 17Cl35	35 – 17 = 18	17	17	2,8,7
Calcium 20Ca40	40 – 20 = 20	20	20	2,8,8,2

From the above information an important term in Chemistry is coined: Valency. This is a number denoting the number of electrons present in the last shell of a neutral atom. It is believed that when two atoms of different elements undergo any chemical change, the valency electrons are transferred from one to another.

A chemical bond is described as the force that actually holds the atoms together within a molecule of substances that have undergone a chemical reaction. So why do elements combine or undergo a chemical reaction?

It has long been known that ‘noble’ gases like Neon do not react chemically. A common feature among all noble gases is that they have either 2 or 8 electrons in their last shell or orbit. Similarly all other elements have between 1 and 7 electrons in their last shell. In 1918 Kossel and Lewis used these assumptions and independently came to the conclusion that a duplet (two electrons in the last shell) or an octet (eight electrons in the last shell), were the most stable configuration for atoms. They further stated in this configuration an atom will be in a minimum state of energy.

Based on this assumption it follows that each atom tries to attain a configuration of duplet or octet in its last shell. Those with one electron in the last shell find it easier to get rid of that electron and those with seven in their last shell find it easier to acquire a single electron. This giving and taking of electrons is what determines whether two elements combine chemically or not. It can further be concluded that all atoms aspire to be ‘noble’ or attain chemical stability by trying to acquire an electronic configuration similar to that of a noble gas. To understand this further the Figure 2.2 shows the electronic configuration of the first 20 elements appearing in the Periodic Table.

Figure 2.2
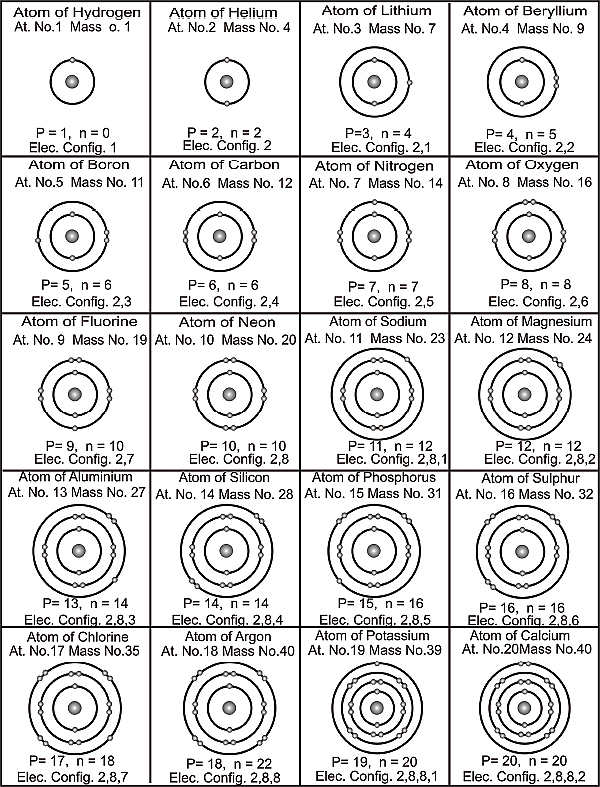
Electronic configuration of atoms

When an atom gives up an electron it acquires a positive charge (+) and when it acquires an electron it becomes negatively charged (–). This can be illustrated with a simple example.

Na – 1e– = Na+
Cl + 1e– = Cl–

In both the examples a stable configuration of 8 electrons (in the last orbit) is obtained. This is the reason why Sodium (Na) and Chlorine (Cl) react vigorously to produce Sodium Chloride (NaCl). The transfer of electrons from one atom to another gives rise to another important term often used in chemistry: Chemical Bond.

In the periodic table the elements are listed in a prescribed format. Besides the obvious grouping of atomic weights the other major criterion used is the number of electrons in the last shell. This is why Lithium, Sodium, and Potassium, appear in the same column (each has one electron in its last orbit).

It is now evident why elements react and why Sodium and Potassium will not react chemically as both of them want to get rid of an electron to obtain stability. It can therefore be concluded that a chemical reaction will most likely take place between an element that wants to get rid of an electron and one that wants to receive an electron. In the example stated above Sodium wants to give up an electron and Chlorine wants to receive an electron. This is the reason that when these two elements come into contact with each other they react vigorously.

2.3 Balancing chemical equations

The first step to balancing equations is to write the chemical components in the form that they exist in nature symbolically. A balanced chemical equation follows the Law of Conservation of Mass and hence to deem an equation balanced the amount of each element reacting must give rise to an equal amount of the same element in the new formed compound. This must be done before the equation can be used in a chemically meaningful way.

A balanced equation has equal numbers of each type of atom on each side of the equation. The Law of Conservation of Mass is the rationale for balancing a chemical equation. Here is the example equation for this lesson:

H2 + O2 ---> H2O

It is an unbalanced equation (sometimes also called a skeleton equation). This means that there are unequal numbers on at least one atom on each side of the arrow.

In the example equation, there are two atoms of hydrogen on each side, but there are two atoms of oxygen on the left side and only one on the right side.

A balanced equation must have equal numbers of each type of atom on both sides of the arrow.

An equation is balanced by changing coefficients in a somewhat trial-and-error fashion. It is important to note that only the coefficients can be changed, never, a subscript. The coefficient times the subscript gives the total number of atoms.

 

Sample problem

As a sample exercise, consider the equation given below. To determine whether this reaction is balanced you must first determine how many atoms of each type are on the reactant side (left-hand side) of the equation and how many atoms of each type are on the product side (right-hand side).

In this example, you have two nitrogen atoms and two hydrogen atoms on the reactant side but only one nitrogen atom and three hydrogen atoms on the product side. For balancing the equation, we are not concerned what molecules these atoms are in, just the number of atoms of each type.

To balance this reaction, it is best to choose one kind of atom to balance initially. Let’s choose nitrogen in this case. To obtain the same number of nitrogen atoms on the product side as on the reactant side requires multiplying the number of product NH3 molecules by two to give:

As you can see above, once we know what the molecules are (N2, H2, and NH3 in this case) we cannot change them (only how many of them there are). The nitrogen atoms are now balanced, but there are six atoms of hydrogen on the product side and only two of them on the reactant side. The next step requires multiplying the number of hydrogen molecules by three to give:

As a final step, make sure to go back and check whether you indeed have the same number of each type of atom on the reactant side as on the product side. In this example, we have two nitrogen atoms and six hydrogen atoms on both sides of the equation. We now have a balanced chemical equation for this reaction

2.4 Chemical periodicity

Lothar Meyer and Dimitri Mendeleev both discovered meaningful patterns of properties among the approximately 63 elements known in 1865.Both listed the elements in order of increasing atomic weight and saw that the properties repeat, a phenomenon called periodicity.

Mendeleev offered some bold, but correct, proposals about places in the scheme that seemed inconsistent and so is generally given credit for the development of the periodic table. Mendeleev’s periodic table left holes where a known element would not properly fit. The classic example is germanium, which was unknown. There was no element in the group that fits the properties of the element below silicon and to the left of arsenic. Mendeleev left that position empty and proposed that the element that belonged there, which he called eka-silicon, was simply yet to be discovered. Within a few years, it was found and its properties matched Mendeleev’s predictions almost perfectly.

At the time of Mendeleev, scientists did not know about the structure of the atom and about subatomic particles and so they did know about atomic numbers. It is a universal fact that the atomic number is the number of protons in the nucleus and therefore it is the charge of the nucleus. The periodic table is actually arranged in order of increasing atomic number, not increasing atomic weight.

A periodic table is included in Appendix -A for reference.

2.4.1 Ionization energy

The ionization energy, IE, is the energy required to remove the outermost electron from a gaseous atom or ion. The first ionization energy, IE1, is the energy for the removal of an electron from a neutral, gaseous atom: M (g) M(g)+ + e. Metallic atoms tend to lose enough electrons to gain the electron configuration of the proceeding noble gas.

There are periodic trends in the ionization energies, also tied to the effective nuclear charge. As the effective nuclear charge increases, it requires more energy to remove the outermost electron from an atom. Consequently, ionization energy is also related to the atomic radius, with ionization energy increasing as atomic radius decreases. Therefore, the first ionization energy increases from left to right in a period and from bottom to top in a group.

2.4.2 Electron affinities

Electron affinity, E, is the energy change of the reaction of adding an electron to a gaseous atom or ion.: M(g) + e M(g)-. These reactions tend to be exothermic and so the values of E are generally negative.

In general, electron affinity tends to decrease (become more negative) from left to right in a period. Going down a group, there is little change in the electron affinities. Negative electron affinity means that the atom gains electrons easily.

2.4.3 Sizes of ions

Recall that atoms increase in size going from right-to left on a period and top-to-bottom in a group.

Cations are smaller than their parent atom because the effective nuclear charge on the outermost electrons is greater in the cation. The number of protons remains the same but the number of screening electrons decreases.

Anions are larger than their parent atoms because the effective nuclear charge on the outermost electrons in smaller in the anion. The number of protons remains the same but the number of screening electrons increases.

Isoelectronic series are groups of atoms and ions which have the same electronic configuration. Within isoelectronic series, the more positive the charge, the smaller the species and the more negative the charge, the larger the species.

Having understood the fundamental way in which atoms react we will now look at how a chemical engineer uses this information to determine reactions, quantities and product values in the field.

2.5 Molecular weight

The molecular weight of a substance is the weight in atomic mass units of all the atoms in a given formula.

An atomic mass unit is defined as 1/12 the weight of the carbon-12 isotope. The old symbol was amu, while the most correct symbol is u (a lower case letter u).

Carbon-12 is defined as weighing exactly 12 amu. This is the starting point for how much an atom weighs.

The molecular weight of a substance is needed tell us how many grams are in one mole of that substance.

The mole is the standard method in chemistry for communicating how much of a substance is present.

The four steps used to calculate a substance’s molecular weight are mentioned below:

Step One: Determine how many atoms of each different element are in the formula.
Step Two: Look up the atomic weight of each element in a periodic table.
Step Three: Multiply step one times step two for each element.
Step Four: Add the results of step three together and round off as necessary.

2.6 The mole and molar mass

The mole is the standard method in chemistry for communicating how much of a substance is present.

Here is how the International Union of Pure and Applied Chemistry (IUPAC) defines “mole:”

The mole is the amount of substance of a system which contains as many elementary entities as there are atoms in 0.012 kilogram of carbon-12. When the mole is used, the elementary entities must be specified and may be atoms, molecules, ions, electrons, other particles, or specified groups of such particles.

One mole contains as many entities as there are in 12 grams of carbon-12 (or 0.012 kilogram). There are 6.022 x 1023 atoms in 12 grams of carbon-12. This number has been very carefully measured in a number of ways over many decades.

 

Avogadro’s number

6.022 x 1023 is so important that it has a name. It is called Avogadro’s number and has the symbol N. It is so named in honor of Amedeo Avogadro, an Italian chemist, who, in 1811, made a critical contribution (recognized only in 1860 after his death) which helped greatly with the measurement of atomic weights.

2.7 Percent composition

Percent composition is the percent by mass of each element present in a compound.

Consider Water (H2O) for an example.

One mole of water is 18.0152 grams.

In that compound, there are two moles of H atoms and 2 x 1.008 = 2.016 grams. That’s how many grams of hydrogen are present in one mole of water.

There is also one mole of oxygen atoms weighing 16.00 grams in the mole of water.

To get the percentage of hydrogen, divide the 2.016 by 18.015 and multiply by 100, giving 11.19%.

For oxygen it is 16.00 ÷ 18.015 = 88.81%.

2.7.1 Molar ratios

The molar ratio will assume a place of central importance in solving stoichiometric problems. The sources for these ratios are the coefficients of a balanced equation.

Consider a sample equation:

2 H2 + O2 ---> 2 H2O

What is the molar ratio between H2 and O2?

  Answer: two to one. So this ratio in fractional form is: 2 / 1

What is the molar ratio between O2 and H2O?

  Answer: one to two. As a fraction, it is: 1/ 2

2.7.2 Mole-mole problems

The procedure used below involves making two ratios and setting them equal to each other. This is called a proportion. One ratio will come from the coefficients of the balanced equation and the other will be constructed from the problem. The ratio set up from data in the problem will almost always be the one with an unknown in it.

It is then possible to cross-multiply and divide to get the answer.

Consider the equation:

N2 + 3 H2 ---> 2 NH3

Problem: If we have 2.00 mol of N2 reacting with sufficient H2, how many moles of NH3 will be produced?

Solution

The ratio from the problem will have N2 and NH3 in it.

How is it possible to know which number goes on top or bottom in the ratios? Answer: it does not matter, except that you observe the next point all the time.

When making the two ratios, be 100% certain that numbers are in the same relative positions. For example, if the value associated with NH3 is in the numerator, then MAKE SURE it is in both numerators.

Use the coefficients of the two substances to make the ratio from the equation.

Why isn’t H2 involved in the problem? Answer: The word “sufficient” removes it from consideration.

Let’s use this ratio to set up the proportion: NH3/ N2
That means the ratio from the equation is: 2 / 1
The ratio from the data in the problem will be: x / 2
The proportion (setting the two ratios equal) is: x / 2 = 2 / 1
Solving by cross-multiplying gives x = 4.00 mol of NH3 produced.

2.7.3 Mole-mass problems

The solution procedure used below involves making two ratios and setting them equal to each other. This is called a proportion. One ratio will come from the coefficients of the balanced equation and the other will be constructed from the problem. The ratio set up from data in the problem will almost always be the one with an unknown in it.

It is now possible to cross-multiply and divide to get the answer.

However, there is one addition to the above technique. One of the values will need to be expressed in moles. This could be either a reactant or a product. In either case, moles will have to convert to grams or the reverse.

Suppose a specific mass is indicated in a problem. It is required to convert this to moles.

Example - If 80.0 grams of O2 was produced, how many moles of KClO3 decomposed?

The ratio from the above statement is: 3/ 2
The ratio from the data in the problem will be: 2.5 / x
The 2.50 mole came from 80.0 g ÷ 32.0 g/mol. The 32.0 g/mol is the molar mass of O2.
Be careful to keep in mind that oxygen is O2, not just O.
Solving by cross-multiplying and dividing gives x = 1.67 mol of KClO3 decomposed.

2.7.4 Mass-mass problems

This is the most common type of stoichiometric problem. There are four steps involved in solving these problems:

• Make sure calculations are based on a properly balanced equation.
• Convert grams of the substance given in the problem to moles.
• Construct two ratios - one from the problem and one from the equation and set them equal. Solve for “x,” which is usually found in the ratio from the problem.
• Convert moles of the substance just solved for into grams.

Remarks

• Double check the equation. Lots of students go right ahead and solve using the unbalanced equation supplied in the problem (or test question for that matter).
• DON’T use the same molar mass in steps two and four.
• Don’t multiply the molar mass of a substance by the coefficient in the problem BEFORE using it in one of the steps above. For example, if the formula says 2 H2O, DON’T use 36.0 g/mol, use 18.0 g/mol.
• Don’t round off until the very last answer.

A graphical representation is given below.

Figure 2.3
Mass-Mass Conversion Chart

2.8 Introduction to solutions

A solution is a particular type of mixture. Mixtures in chemistry are combinations of different substances where each substance retains its chemical properties. Generally, mixtures can be separated by non-chemical means such as filtration, heating, or centrifugation.

A solution is a homogeneous mixture where all particles exist as individual molecules or ions. There are homogeneous mixtures where the particle size is much larger than individual molecules. However, the particle size is so small that the mixture never settles out. Terms such as colloid, sol, and gel are used to identify these mixtures.

A solution has two components: the solute and the solvent.

The solvent is the substance in greater amount.

It is usually a liquid, although it does not have to be. It is usually water, but it does not have to be. We will focus on water only and will leave non-aqueous solvents alone.

The solute is the substance in lesser amount.

 

Molarity

The molarity of a solution is calculated by taking the moles of solute and dividing by the liters of solution.

Dilution: Definition and Calculations

To dilute a solution means to add more solvent without the addition of more solute. Of course, the resulting solution is thoroughly mixed so as to ensure that all parts of the solution are identical. The fact that the solvent amount stays constant allows us to develop calculation techniques.

Moles before dilution = moles after dilution

From the definition of molarity, the moles of solute equals the molarity times the volume.

Hence it is now possible to substitute MV (molarity times volume) into the above equation, like this:

M1V1 = M2V2

In the following sections we will review the other measurements that a chemical engineer uses in a process plant.

2.9 Units and dimensions

A measured or numbered quantity has a numerical value and a unit associated with it. It is useful and essential in most engineering calculations to specify both the parameters appearing in an equation. A dimension is a property that can be measured, such as length, time, mass or temperature. Alternatively, it is obtained by multiplying or dividing other dimensions; such as length/time (velocity), length3 (volume), or mass/length3 (density). Measurable units are specific values of dimensions that are outlined by conventions, custom or law, such as mass (grams), time (seconds), or feet (centimeters) etc. Units are similar to algebraic variables when quantities are added, subtracted, multiplied or divided. The numerical values of two quantities may be added or subtracted only if the units are the same. Numerical values and their corresponding units can be combined by multiplication or division.

2.9.1 Systems of units

A system of units comprises the following components:

Base units or units

These units indicate the dimensions of mass, length, temperature, time, electrical current and light intensity.

Multiple units

They are defined as multiples or fractions of base units such as minutes, hours and milliseconds, all of which are defined in terms of the base unit of a second. Multiple units are defined for convenience rather than necessity.

Derived units

They are obtained in one of two ways:

• By multiplying and dividing base or multiple units (cm2, ft/min, kg m/s2, etc). Derived units of this type are commonly referred to as compound units
• As defined equivalents of compound units (e.g. 1 erg = 1g cm/s2)

During the 1960’s, an international conference proposed a system of metric units that is widely accepted in the scientific and engineering field. It is known as the “Systeme Internationale d’Unites,” or the SI system for short. Unit prefixes are used in the SI system to indicate powers of ten. The CGS (Centimeter, Gram, Second) system is almost identical to the SI system; the main difference being that grams (g) and centimeters (cm) are used instead of kilograms and meters as the base units of mass and length. The base units of the American engineering system are the foot (ft) for length, the pound mass (lbm) for mass and the second (s) for time.

2.9.2 Conversion of units

A measured quantity can be defined in terms of any units having the suitable dimensions. The equivalence between two expressions of a given quantity may be expressed in terms of a ratio. For example, velocity can be expressed in terms of ft/sec, miles/hr, or any other ratio of a length unit to a time unit. The numerical value of the velocity then is based on the unit chosen.

Ratios as described above are known as conversion factors.

To convert a quantity expressed in terms of one unit to its equivalent in terms of another unit, multiply the given quantity by the conversion factor (new unit /old unit).

2.9.3 Force and weight

Newton’s second law of motion states that force is proportional to the product of mass and acceleration (length/time2). Natural unit of force is therefore, kg m/s2 (SI), g cm/s2 (CGS) and lbm. ft/s2 (American engineering).

The equation which connects force in defined units to mass and acceleration is

F = ma / gc

The weight of an object is the force exerted on the object by the gravitational attraction of the earth. Consider an object of mass m, is subjected to a gravitational force W (W is by definition the weight of the object). If this object were falling freely its acceleration would be g. The weight, mass and free fall acceleration of the object are related by the following equation:

W = mg / gc

The value of g at sea level and 450 latitude and corresponding value of g/gc are given below in each system of units:

g = 9.8066 m/s2 => g/gc = 9.8066 N/kg

g = 980.66 cm/s2 => g/gc = 980.66 dyne/g

g = 32.174 ft / s2 => g/gc = 1 lbf / lbm

2.9.4 Dimensional homogeneity and dimensionless quantities

Every valid equation must be dimensionally homogeneous which means, all additive terms on both sides of the equation must have the same units.

A dimensionless quantity can be a pure number (e.g. 2, 3.5) or a multiplicative combination of variables with no net units:

M (g) / M0 (g)

Quantity like (M/M0) is called a dimensionless group.

Certain important dimensionless numbers are mentioned below.

• Archimedes Number
• Arrhenius Number
• Bingham Number
• Capillary Number
• Cavitation Number
• Darcy friction factor
• Drag Coefficient
• Elasticity Number
• Fourier Number
• Heat Transfer Factor
• Mass Transfer Factor
• Reynolds Number

2.9.5 Arithmetic calculations

• A rule of thumb is that when two or more quantities are combined by multiplication and/or division, the number of significant figures in the result should equal the lowest number of significant figures of any of the multiplicants or divisors
• The rule is that when two or more numbers are added or subtracted, the positions of the last significant figures of each number should be compared. Of these positions, the one farthest to the left is the position of the last permissible significant figure of the sum
• The logic behind rounding off numbers in which the digit to be dropped is a 5, is always to make the last digit of the rounded-off number even

e.g. 2.68 => 2.7, 5.34 =>5.3

2.10 Process variables

A process is any operation or series of operations that results in a physical or chemical change in a material or a mixture of materials. The material that enters a process is referred to as the input or feed to the process and that which leaves is called the output or product. A process unit is a device in which one of the operations that constitutes a process is carried out. Each process unit has associated with it a set of input and output process streams, which consists of the materials that enter and leave the unit.

The process variable is a set of quantities that defines the operating conditions of a reactor or a system of reactors.

2.10.1 Mass and volume

The density of a substance is the mass per unit volume of the substance. The specific volume of a substance is the volume per unit mass of the substance and is therefore the inverse of the density. Densities of pure solids and liquids are relatively independent of temperature and pressure and may be found in standard references (such as the Chemical Engineers’ Handbook).

The specific gravity (SG) of a substance is the ratio of the density ρ of the substance to the density ρref of a reference substance at a specific condition.

SG = ρ / ρref

2.10.2 Flow rate

Mass and volumetric flow rate

Continuous processes involve the movement of material from various process units. The rate, at which material is transported through a process line, is the flow rate of that material.

The flow rate of a process stream may be expressed as a mass flow rate (mass/time) or as a volumetric flow rate (volume/time). Consider a fluid (gas or liquid) which flows in a cylindrical pipe as shown in Figure 2.5, where the shaded area represents a section perpendicular to the direction of flow.

If the mass flow rate of the fluid is m (kg/sec), then every second m kilograms of the fluid pass through the cross section. If the volumetric flow rate of the fluid at the given cross-section is V (m3/sec), then every second V cubic meters of the fluid pass through the cross section. However, the mass m and the volume V of a fluid (in this case, the fluid that passes through the cross section each second) are not independent quantities but are related through the fluid density ρ:

ρ = m / V

Figure 2.4
Mass and Volumetric Flow rate

2.10.3 Pressure

Fluid pressure and hydrostatic head

A pressure is the ratio of a force to the area on which it acts. Accordingly, pressure units are force units divided by area units (e.g. N/m2, dynes/cm2....). The SI pressure unit (N/m2) is called a Pascal (Pa).

Consider a fluid (gas or liquid) contained in a closed vessel or flowing through a pipe and suppose that a hole of area A is made in the wall of the containing vessel, as in Figure 2.6. The fluid pressure may be defined as the ratio (F/A), where F is the minimum force that would have to be exerted on a plug in the hole to keep the fluid from emerging.

Figure 2.5
Fluid Pressure in Tank and a pipe

Consider a vertical column of fluid is h meters high and has a uniform cross sectional area A(m2) Fig 3.3. Further, assume that the fluid has a density of ρ(kg / m3) and that a pressure po (N/m2 ) is exerted on the upper surface of the column. The pressure P of the fluid at the base of the column is called the hydrostatic pressure of the fluid (F/A). F thus equals the force on the top surface plus the weight of the fluid in the column.

P = P0 + ρ(g/gc) h

Figure 2.6
Pressure at the Base of a Fluid Column

2.10.4 Temperature

The temperature of a substance in a particular state (solid, liquid or gas) is a measure of the average kinetic energy held by the molecules.

The two most common temperature scales are defined using the freezing point (Tf ) and boiling point (Tb) of water at a pressure of 1 atmosphere.

Celsius (or centigrade) scale: Tf is assigned a value of 0°C and Tb is assigned a value of 1000C. Absolute zero (theoretically the lowest temperature that can be reached in nature) on this scale falls at -273.15°C.

Fahrenheit scale: Tf is assigned a value of 32°F and Tb is assigned a value of 212°F. Absolute zero falls at –459.67°F.

The Kelvin and Rankine scales are defined such that absolute zero has a value of 0 and the size of a degree is the same as a Celsius degree (Kelvin scale) or a Fahrenheit degree (Rankine scale).

The following relationships may be used to convert a temperature expressed in one defined scale unit to its equivalent in another.

T(K) = T(°C) + 273.15

T(°R) = T(°F) + 459.67

T(°R) = 1.8T(K)

T(°F) = 1.8T(°C) + 32

A degree is to denote both a temperature and its interval.

Consider the temperature interval from 0°C to 5°C. There are nine Fahrenheit and Rankine degrees in this interval and only five Celsius and Kelvin degrees. An interval of 1 Celsius or Kelvin degree therefore contains 1.8 Fahrenheit or Rankine degrees, leading to these conversion factors:

1.8°F / 1°C

1.8°R / 1K

1°F / 1°R

1°C/ 1K